1 Plus 1 Equals 4

Mathematical space series

First six summands drawn as portions of a square.

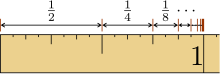
The geometric serial on the existent line.

In mathematics, the infinite serial 1 / 2 + 1 / 4 + 1 / eight + 1 / sixteen + ··· is an simple instance of a geometric serial that converges admittedly. The sum of the series is 1. In summation notation, this may be expressed as

${\displaystyle {\frac {1}{ii}}+{\frac {1}{four}}+{\frac {1}{8}}+{\frac {1}{16}}+\cdots =\sum _{n=ane}^{\infty }\left({\frac {1}{two}}\right)^{north}=i.}$

The series is related to philosophical questions considered in antiquity, especially to Zeno's paradoxes.

Proof [edit]

As with whatsoever infinite series, the sum

${\displaystyle {\frac {i}{2}}+{\frac {1}{4}}+{\frac {1}{8}}+{\frac {i}{16}}+\cdots }$

is divers to mean the limit of the partial sum of the offset n terms

${\displaystyle s_{northward}={\frac {one}{ii}}+{\frac {1}{4}}+{\frac {1}{8}}+{\frac {1}{16}}+\cdots +{\frac {i}{2^{n-1}}}+{\frac {1}{2^{n}}}}$

as n approaches infinity. Past various arguments,^[a] 1 can evidence that this finite sum is equal to

${\displaystyle s_{n}=1-{\frac {1}{2^{n}}}.}$

Equally due north approaches infinity, the term ${\displaystyle {\frac {1}{2^{n}}}}$ approaches 0 and then southward_north tends to one.

History [edit]

Zeno'southward paradox [edit]

This serial was used as a representation of many of Zeno'due south paradoxes.^[i] For example, in the paradox of Achilles and the Tortoise, the warrior Achilles was to race confronting a tortoise. The track is 100 meters long. Achilles could run at 10 chiliad/s, while the tortoise only 5. The tortoise, with a 10-meter reward, Zeno argued, would win. Achilles would have to move 10 meters to catch upwards to the tortoise, but the tortoise would already have moved some other v meters by and then. Achilles would then have to movement 5 meters, where the tortoise would motility 2.five meters, and so on. Zeno argued that the tortoise would ever remain ahead of Achilles.

The Dichotomy paradox also states that to motility a sure distance, y'all have to move half of it, and so half of the remaining distance, and and then on, therefore having infinitely many time intervals.^[1] This can be easily resolved by noting that each time interval is a term of the space geometric series, and will sum to a finite number.

The Centre of Horus [edit]

The parts of the Centre of Horus were one time thought to represent the first six summands of the series.^[2]

In a myriad ages it volition not be exhausted [edit]

A version of the series appears in the ancient Taoist book Zhuangzi. The miscellaneous chapters "All Under Sky" include the following sentence: "Take a chi long stick and remove half every mean solar day, in a myriad ages it volition non be wearied."^{[ citation needed ]}

Encounter also [edit]

• 0.999...
• one/two − 1/4 + one/8 − 1/xvi + ⋯
• Actual infinity

Notes [edit]

References [edit]

1. ^ ^{a b} Field, Paul and Weisstein, Eric Due west. "Zeno'south Paradoxes." From MathWorld--A Wolfram Spider web Resource. https://mathworld.wolfram.com/ZenosParadoxes.html
2. ^ Stewart, Ian (2009). Professor Stewart's Hoard of Mathematical Treasures. Contour Books. pp. 76–80. ISBN978 1 84668 292 half dozen.

1 Plus 1 Equals 4,

Source: https://en.wikipedia.org/wiki/1/2_+_1/4_+_1/8_+_1/16_+_%E2%8B%AF

Posted by: turnerentinver.blogspot.com

Share this post